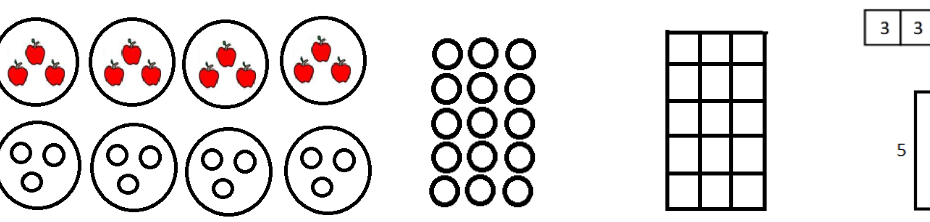Skip to content
Home » Blog » Concrete-Pictorial-Abstract Learning Progression

Concrete-Pictorial-Abstract Learning Progression

Representations of the equation five times three equals fifteen including equal groups with objects, equal groups drawn with circles, arrays, a rectangle with a grid, a tape diagram, and an area model with the side lengths labeled.

Concrete, pictorial, and abstract representations should be central to every mathematics classroom. No matter what grade level you teach, these three words are the key to building students’ conceptual understanding and confidence and providing equitable access to grade-level content. Students who clearly understand abstract notation means can connect and generalize knowledge across concepts and situations more easily.

Concrete:

Concrete refers to objects students can physically touch, move, hold, or manipulate. Concrete materials allow students to see the properties of operations in action. They help students think about proportionality and the effects of each mathematical operation. Concrete objects allow students to explore and discover how numbers work. For example, representing multiplication with concrete objects shows students how one factor identifies the number of groups and the second factor determines the size of each group. So, students make five groups of three to represent 5 x 3. 

A student created five groups of three linking cubes to show five groups of three or five times three.

Pictorial:

Pictorial or semi-concrete representations are the umbrella term for any drawn models. It is essential to recognize that there are many levels of pictorial representations. 

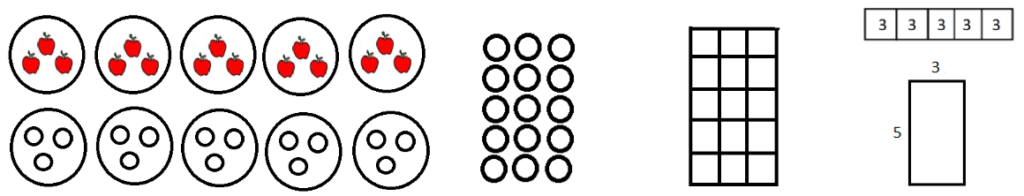
This image shows a progression of six pictorial representations of five groups of three. The first image shows five circles with three apples in each. In the second image instead of apples there are simple open circles. The third image shows an array of open circles with five rows, each row has three columns. Next to that array is a rectangle with a grid showing five rows of three square units. The last two drawings are the most abstract. There is a tape diagram/bar model showing a rectangle broken into five equal parts. Each part is labeled with the number 3. There is also a rectangle where the side lengths are labeled. The length is 5 units and the width is 3 units.

Pictures can be representational when students create drawings similar to the objects they represent. For example, students might draw actual apples to illustrate a story problem about picking apples. As students move toward abstraction, the next step is to represent objects with a more simplified drawing, such as an open or closed circle or an x. The pictorial models in the image above become increasingly more abstract from left to right. The first drawing, an equal groups model, represents each group in a separate space or circle. Next, you see arrays. In the first array, the red dots are arranged in rows where each row represents one group. The second array is more visually complex because the grid has no space between the square units, but students still see three units in each row. The final drawings are the tape diagram or bar model and an area model. These are the most abstract because the number of items in each group is solely represented by the number 3 in the bar model, and the side lengths represent the number of groups and the size of each group in the area model. Students need to understand and visualize the set of three this number represents.

Abstract:

An abstract representation is when mathematical notation is solely used to represent a student’s thinking. Usually, abstract notation consists of numbers, mathematical symbols, and sometimes letters for variables. This is the most complex way students represent mathematical thinking. If students use abstract notation to show their thinking, they must understand the meaning of that notation deeply enough to clearly explain or draw a picture to show their thinking process.  

This image shows two equations. The first equation is three plus three plus three plus three plus three equals fifteen. The second equation is five times three equals fifteen.

These two equations show increasingly abstract ways to represent the thinking in multiplication. The first equation shows repeated addition. In this equation, a student can see each of the five groups of three being added together to find the total. In the second equation, the first factor tells us to picture 5 groups, and the second factor tells us that each group has three for a total product of 15.

Concrete-Pictorial-Abstract is Not a Linear Progression

Ideally, every student experiences new content beginning with a concrete representation. As students progress through the learning progression for a concept, they may switch back and forth between concrete, pictorial, and abstract representations. When new units or larger numbers are introduced, students may need to return to pictorial or concrete representations to maintain deep conceptual understanding. One example of this may occur between two of the pictorial representations above.

This image shows five circles that each had three red cubes. In the picture the three cubes from the first circle have been moved into a row of three next to the equal groups drawing. The second group of three cubes has also been moved into a row of three cubes underneath the first row. One cube from the third equal group has been moved into the first spot in the third row. The remaining two cubes of the third group and the last two groups of three cubes are still in equal groups. Next to the partial array of cubes is a drawing of an array showing five rows of three open circles.

This image is a sequence that might occur after students have moved from making equal groups with objects to drawing equal groups. When students are introduced to arrays, a new way to represent equal groups, the teacher might have students go back to concrete objects. First, students can make equal groups with cubes, a skill they have mastered. Then, moving the cubes from each group into a row allows students to create a new model with concrete objects first. Once they experience this using hands-on manipulatives, the next step is for students to create a drawing to show the array they made with cubes. The one new thing in this moment is an array. Returning to concrete objects between the equal groups and array drawings helps ensure that students understand how the two representations are connected.

Concrete-Pictorial-Abstract for Access and Equity

Understanding the concrete-pictorial-abstract progression can be the key to access and equity. If students can move fluidly back and forth in this progression, all students can use what they know to access grade-level content. If students in your class are missing some foundational knowledge for a concept you are teaching, concrete objects might provide them an access point. If students struggle with a new complexity presented in a lesson abstractly, a pictorial representation might be all they need to make sense of it. The key to leveraging this progression is remembering, before reducing the complexity in the numbers try using a more scaffolded model.

Written by LauraMarie Coleman, January 28, 2025

Tags: