I recently had the opportunity to meet with a fourth-grade teacher to review student work from her most recent formative assessment. When the teacher entered the room, she slumped in the chair and shared her defeated declaration: “Only 3 of my students got this right.” As a teacher, I can identify with this level of frustration and exhaustion. As an instructional coach, I saw an opportunity to help this teacher see her class in a new light.
The teacher shared the supports and scaffolds her class had used during the lessons on this topic before the quiz. Then, I suggested we go through the student work together. My one condition was that we only look for what each student knew about equivalent fractions.
A Move to Asset-Focused Analysis
Once she agreed, we both jumped into analyzing the students’ thinking. On the first paper, we saw evidence that the student understood that two equivalent fractions would line up when they used double number lines. The following student’s work showed a similar understanding. Before we knew it, we identified that all but one student in her class understood how the double number lines helped them find an equivalent fraction. Their struggle was in how to create the number lines.
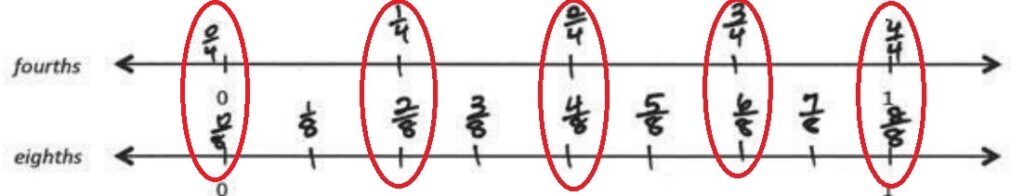
As we continued to examine the student work for the second problem, it quickly became apparent that many students understood that they could use multiplication to find an equivalent fraction, too. Like the double number lines, students used the information they had learned. The problem with this strategy was that they didn’t understand why it worked.
Deficit thinking digs a hole; Asset thinking defines a path.
Once we analyzed the students’ work, the teacher had a three-step to-do list for her upcoming lessons. We identified four students who needed intensive support on the meaning of the numerator and denominator. Most of the class required explicit instruction and opportunities to practice creating number lines for fractions. The whole class needed explicit instruction on how the partitioning of the double number lines is directly connected to the thinking when students use multiplication to find equivalent fractions.
By the end of our meeting together, the teacher left excited about today’s math lesson. Despite most students getting the wrong answers on the formative assessment, she felt confident that her students were making a lot of progress in their understanding of equivalent fractions. She also knew that most of them were very close to having two valuable strategies to help them with this work.
Identifying what students know gives us a place to start.
Learning something new is always a process. At times, the early stages of any learning process can feel overwhelming. When students first explore something new and try to use what they know in a new context or expand their conceptual understanding, it often feels like we get more wrong than right. As teachers, we must focus on growth and not perfection when looking at formative assessment data. When we help students see and stay focused on their foundational knowledge growing into new understandings, every step of the learning process feels like a great accomplishment.
